A value that on multiplication by itself gives the original number is known as the square root of a number. Square and square root are related topics. An inverse method of squaring a number is the square root of a number. Let us take an example of a square root, the square of 3 is 9, and the square root of 9 is 3. Since 9 is a perfect square, the square root is easy to find. We have been studying square roots since our childhood in our schools, and it has been one of the most interesting topics. In this article, we will try to understand some topics related to square roots, such as the symbol of square root, its properties, and see some square roots of perfect squares.
What is a Cube Root?
A value that on multiplication by itself gives thrice or three times the value of the original number is called cube root. For example, the cube root of 27 is 3, which is denoted by the symbol 3√27. Since 27 is a perfect cube, the cube root is easy to find. In three-dimensional geometry, when we learn about the different solids, the cube of an object defines which has all its faces or sides equal in dimension. If you want to learn more about square roots and cube roots in a fun and interactive manner, visit cuemath.com and learn from the best tutors of mathematics who lay emphasis on concept clarity.
Symbol of Square Root
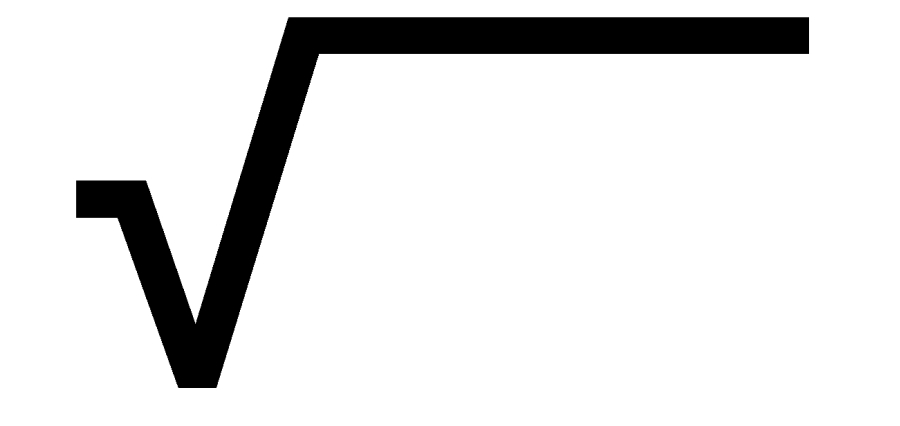
The symbol of the square root is usually denoted by √. It is known as a radical symbol. To represent a number, let say x as a square root; we can write √x. For example, the square root of 9 is three; in mathematical terms, we can write as √9 is equal to 3. The number under the radical symbol is known as the radicand.
Properties of Square Root
Some of the significant properties of the square root are as follows.
- If a number is a perfect square number, then there exists a perfect square root. Let us understand this in a better way with the help of an example. The square of 5 is 25, which basically means 5 × 5 is equal to 25. Here, 25 is a perfect square number. Thus, the square root is easy to calculate.
- The square root of two numbers can be multiplied to get a suitable result. For example, root 3 multiplied by root 2 is root 6.
- The square root of a number that is negative can never be defined because a perfect square can never be negative.
- If a number ends with the last digit as 2,3,7,8, the perfect square does not exist.
- Similarly, if a number ends with the last digit 1,4,5,6,7,9 in the unit digit, then the number will have a square root.
- When two square roots are multiplied, the result is a radical number. For example, when we multiply √7 by √7 the result is 7. Thus justified.
Some Square Root of Perfect
The following numbers mentioned below are the perfect squares. Finding the square roots of such number is way easier:
1.1 = 1, √1 = 1
2.2 = 4, √4 = 2
3.3 = 9, √9 = 3
4.4 = 16, √16 = 4
5.5 = 25, √25 = 5
6.6 = 36, √36 = 6
7.7 = 49, √49 = 7
8.8 = 64, √64 = 8
9.9 = 81, √81 = 9
10.10 = 100, √100 = 10
Symbol of Cube Root
The cube root symbol is denoted by 3√. In the case of a square root, we have just used the symbol √, which is also called a radical. Therefore, symbolically we can represent the cube root of a different number as the Cube root of X = 3√X.